Convexity and limits in quantum information theory

As quantum science is transformed into a viable technology, it is of increasing importance to identify and develop mathematical techniques that allow a rigorous understanding of quantum information transmission. The identification of the fundamental capabilities and limitations of quantum computing poses hard mathematical problems, intercrossing a number of areas and requiring a variety of techniques from linear algebra, functional analysis, probability theory, operator theory, and others. A feasible passage from the classical to the quantum computer  would rest on fruitful analogies inspired by classical information theory as a sample of physically realisable theoretical patterns, giving rise to a far-reaching cross-fertilisation between this field and quantum information theory. We illustrate these efforts by several examples:

1. Channel capacities are the optimal rates of broadcasting quantum information that leads to a physically reliable transmission. A genuinely quantum effect was discovered in 2009: two quantum channels that have capacity zero of transmitting quantum information perfectly, when  plugged in parallel, may be used to transmit with perfect reliability non-trivial amount of both classical and quantum information. This phenomenon, called superactivation, is linked to a series of problems about the computation of the capacity of channels applied in parallel, in terms of the capacities of the individual channels, and many of its aspects remained unexplained.
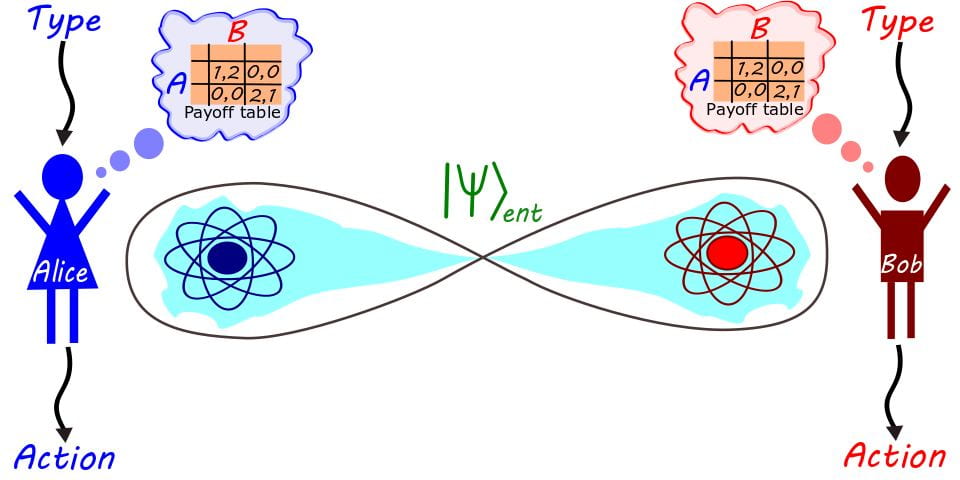
2. Entanglement is one of the most intriguing features of quantum mechanics. Arguably, one of its most spectacular manifestations arises through pseudo-telepathy games: cooperative games played by two players against a third verifying party, which cannot be won via classical strategies, but can be won using strategies relying on quantum entanglement. The usefulness of such games is highest when the game has a very large size. It therefore becomes necessary to develop a mechanism which allows the analysis of large games, both in isolation and in conjunction with other quantum games. The formulation of a mathematical framework of limit games will allow the probabilistic analysis of subtle questions about winning probabilities in the important parallel repetition problem.
3. Convex sets and functions play an indispensable role in analysis, optimization, and many other areas of mathematics and data science. We are exploring a notion of convexity that applies to quantum density operators on a Hilbert space. This arena is largely unexplored, but we expect that once the theory is properly developed, “convex density operators” should play a similarly indispensable role in the study of quantum mechanical systems.

Participating Faculty: Ghandehari, Madiman, Todroov